EJSS simple harmonic motion model with Amplitude vs frequency graphs
based on models and ideas by
 |
EJSS SHM model with resonance showing Amplitude vs frequency graphs, heavy damping (RED)
frequency ratio for better x azes values
https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHMresonance01/SHMresonance01_Simulation.htmllatest: src: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHM24.zip model: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHM24.zip run: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHM24/SHM24_Simulation.xhtml
source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_SHMresonance01.zip
source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_SHMresonance.zip
author: lookang
author of EJSS 5.0 Francisco Esquembre
|
 |
EJSS SHM model with resonance showing Amplitude vs frequency graphs, heavy damping (RED)
frequency ratio for better x azes values
https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHMresonance01/SHMresonance01_Simulation.html
source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_SHMresonance01.zip
source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_SHMresonance.zip
author: lookang
author of EJSS 5.0 Francisco Esquembre
|
- lookang http://weelookang.blogspot.sg/2014/03/ejs-resonance-frequency-vs-amplitude.html
- Wolfgang Christian EJS examples by wolfgang, such as\source\users\davidson\wochristian\osc\SHOResonance.xml
No damping
 |
| EJSS SHM model with resonance showing Amplitude vs frequency graphs, no damping http://weelookang.blogspot.com/2014/03/ejss-shm-model-with-resonance-showing.html https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHMresonance/SHMresonance_Simulation.html source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_SHMresonance.zip author: lookang author of EJSS 5.0 Francisco Esquembre |
Very light damping
 |
| EJSS SHM model with resonance showing Amplitude vs frequency graphs, very light damping (RED) http://weelookang.blogspot.com/2014/03/ejss-shm-model-with-resonance-showing.html https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHMresonance/SHMresonance_Simulation.html source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_SHMresonance.zip author: lookang author of EJSS 5.0 Francisco Esquembre |
Light damping
 |
| EJSS SHM model with resonance showing Amplitude vs frequency graphs, light damping (RED) http://weelookang.blogspot.com/2014/03/ejss-shm-model-with-resonance-showing.html https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHMresonance/SHMresonance_Simulation.html source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_SHMresonance.zip author: lookang author of EJSS 5.0 Francisco Esquembre |
Moderate damping
 |
| EJSS SHM model with resonance showing Amplitude vs frequency graphs, moderate damping (RED) http://weelookang.blogspot.com/2014/03/ejss-shm-model-with-resonance-showing.html https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHMresonance/SHMresonance_Simulation.html source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_SHMresonance.zip author: lookang author of EJSS 5.0 Francisco Esquembre |
Critical damping
 |
| EJSS SHM model with resonance showing Amplitude vs frequency graphs, critical damping (RED) http://weelookang.blogspot.com/2014/03/ejss-shm-model-with-resonance-showing.html https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHMresonance/SHMresonance_Simulation.html source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_SHMresonance.zip author: lookang author of EJSS 5.0 Francisco Esquembre |
Heavy damping
 |
| EJSS SHM model with resonance showing Amplitude vs frequency graphs, heavy damping (RED) http://weelookang.blogspot.com/2014/03/ejss-shm-model-with-resonance-showing.html https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHMresonance/SHMresonance_Simulation.html source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_SHMresonance.zip author: lookang author of EJSS 5.0 Francisco Esquembre |
Wikipedia
 |
| https://upload.wikimedia.org/wikipedia/commons/thumb/0/07/Resonance.PNG/1200px-Resonance.PNG |
YJC note
 |
| yjc notes |
The equations that model the motion of the many spring mass system are:
$ F[i] = - k x[i] $
where $ F[i] $ is the restoring elastic force exerted by the spring (in SI units: N), k is the spring constant (N·m−1), and x[i] is the displacement from the equilibrium position (in m).
Thus, this model assumes an array of object in [i] dimensional where number of objects is n = 50
$ \frac{\delta x[i]}{\delta t} = v_{x}[i] $
$ \frac{\delta v_{x}[i]}{\delta t} = -\frac{k}{m}(x[i]-l) - \frac{bv_{x}[i]}{m} + \frac{A sin(2 \pi f[i] t)}{m} $
where the terms
$ -\frac{k}{m}(x[i]-l) $ represents the restoring force component as a result of the spring extending and compressing.
$ - \frac{bv_{x}[i]}{m}$ represents the damping force component as a result of drag retarding the mass's motion.
$ + \frac{A sin(2 \pi f[i] t)}{m} $ represents the driving force component as a result of a external periodic force acting the mass $ m $.
Forced oscillations
Forced oscillations are oscillations that are subjected to a periodic driving force provided by an external agent such as motor or a push by a person etc.
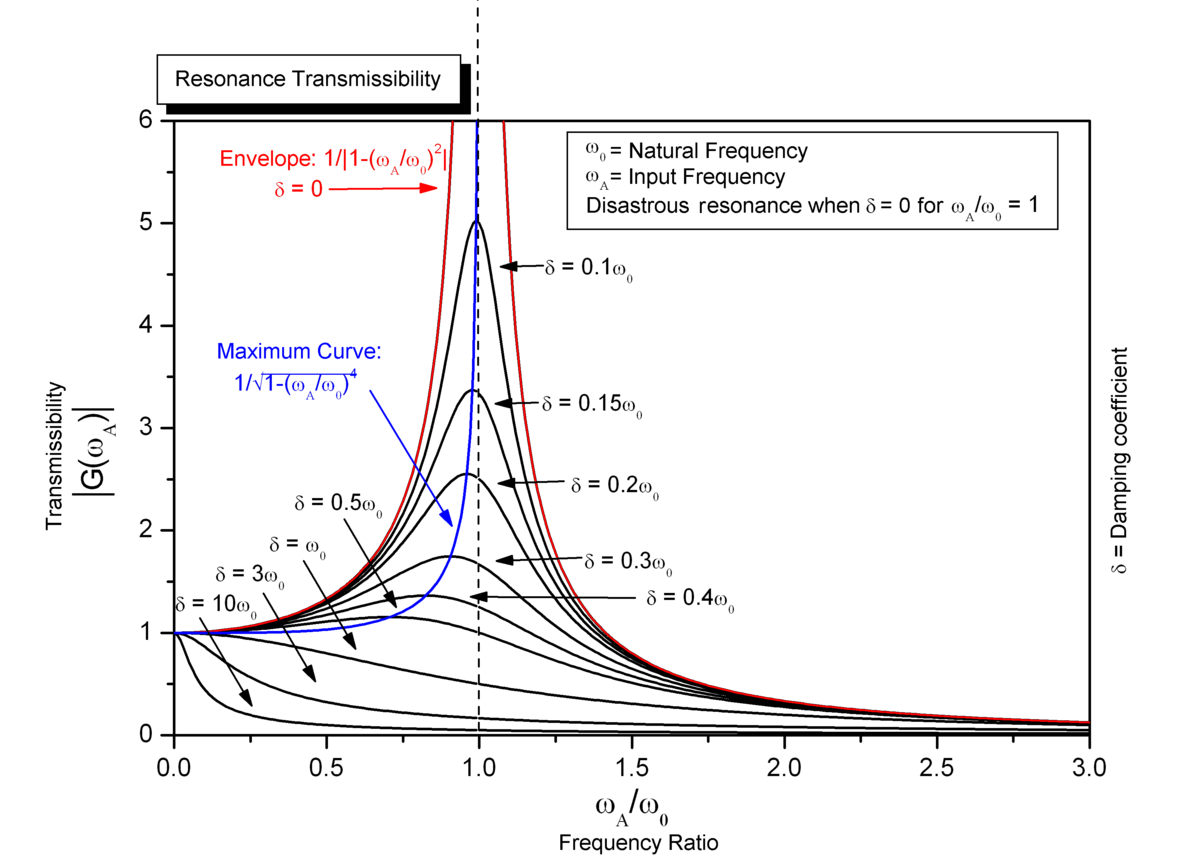
Resonance is an interesting phenomenon that occurs when driving force frequency matches that of the system's natural oscillating frequency resulting in a motion that reaches some maximum amplitude.
Resonance
In physics, resonance is the tendency of a system to oscillate with greater amplitude at some frequencies than at others. Frequencies at which the response amplitude is a relative maximum are known as the system's resonant frequencies, or resonance frequencies. At these frequencies, even small periodic driving forces $ + \frac{A sin(2 \pi f[i] t)}{m} $ can produce large amplitude oscillations, because the system stores vibrational energy.Resonance occurs when a system is able to store and easily transfer energy between two or more different storage modes (such as kinetic energy and potential energy in the case of a spring mass system). However, there are some losses from cycle to cycle, called damping. When damping is small, the resonant frequency is approximately equal to the natural frequency of the system, which is a frequency of unforced vibrations. Some systems have multiple, distinct, resonant frequencies.
No comments:
Post a Comment